Two Oppositely Charged Extremal Black Holes Produced From Nothing
It seems like there is an infinite number of interesting things to say about black holes, which are the final state of collapse for a large number of massive stars. While one black hole is complicated enough, and in science, we typically evoke the solitary image of a single behemoth of fire capable of easily swallowing whole legions of planets; the story becomes much richer when we place two of them in proximity. In astrophysical situations, this is a well-studied and simulated setup known as the two-body problem, which leads to interesting phenomena like mergers, the collision of two worlds and their union into a single whole. In this article, we will provide a different spin on the story of a 'merger' which takes us through the wondrous birth of a pair of accelerating, precisely-matched, and massive black holes with opposite charges in our universe, ending with both of them traveling along to the edge of spacetime.
Einstein and extremes

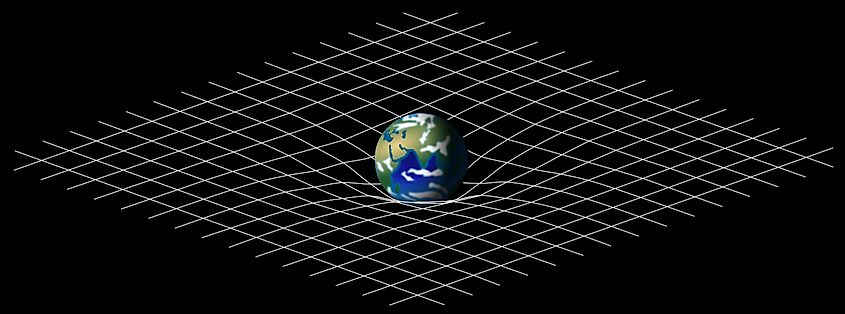
In the early twentieth century, Albert Einstein completely reimagined our understanding not only of gravity but also the very structure of background concepts we take for granted, like space and time. The synthesis of his theory, general relativity, is a fabric that stitches together both space and time in a beautiful pattern dictated by the behavior of matter and energy embedded in it. Colloquially, one says that the influence of matter on space and time results in the bending of spacetime, but a better word might be a distortion or an imprinting. In this mathematical theory that has withstood more than a century of experimental verification, one obtains possible universes by solving the equations Einstein wrote down. One very important class of solutions is black holes, which, in certain scenarios, can be proved to be unique. For example, the Kerr-Newman solution describes the unique black hole solution in four dimensions obeying the following conditions:
- It is stationary, so the universe does not evolve explicitly in time, which justifies calling it the final state of collapse.
- It is asymptotically flat, so one can treat it as an isolated black hole in a single universe.
- It is axisymetric, meaning that the black hole is invariant under rotations about a particular axis and so it has a notion of angular momentum and spin.
- It is labeled by solely three numbers: mass, charge, and angular momentum, characterizing the particular choice of solution in the class.

Since there is a manifold of such black holes given by the combinations of mass, charge, and angular momentum, one may try to understand the special cases in terms of simpler spacetimes. For example, when all the numbers are zero, we get no black hole, and the universe is empty. When there is no angular momentum, which is the case we will be interested in, there will be a special black hole family characterized by a single parameter instead, where one enforces a relationship between the mass and charge. Such solutions are called extremal and play a fundamental role in the study of black hole physics because of their shocking simplicity yet enigmatic character. These black holes have the minimum amount of mass to sustain their charge and so could be analogized to the building blocks of black hole physics. It is important to note that while we have never measured such objects, there is considerable mathematical evidence that extremal black holes actually exist as valid solutions of general relativity, which we discussed in a previous article. The nucleation of the pair of black holes from nothing is possible because of the fine-tuning required in the extremality condition of these black holes.
Instantons versus solutions
The charged black hole solutions above are solutions to Einstein's original equations. However, nucleation involves a fundamental concept in physics called an instanton. To understand quantum processes like tunneling or the creation of particle-antiparticle pairs, physicists often use a very nifty trick referred to as Wick rotation or Euclideanization. While space and time are blended together in relativity, time retains its special characteristic of distinguishing between the future and the past, which is parametrized by a negative sign. By replacing time with imaginary time, which leads to an additional negative sign, we end up with geometry in the sense of mathematicians that is purely spatial, otherwise known as Euclidean geometry.

These solutions of the Euclidean version of the classical theory, which we take to be general relativity here, are called instantons. They’re not physical as they do not entertain a valid notion of time, yet they have a lot to say about physics that one could not see very clearly before their introduction. These instantons are responsible for many of the unfamiliar phenomena of quantum theory, such as tunneling, also covered in a previous article. In quantum mechanics, instantons describe processes that are classically forbidden. In gravity, they can describe the very birth of spacetimes of wildly different characters.
Extremal black hole nucleation

In seminal work from just before the turn of this century, two theoretical physicists, David Garfinkle, and Andrew Strominger, argued that if gravity were subject to the same quantum-mechanical laws as atoms, then tunneling from nothing to a pair of black holes should be a possible process. They were able to find an instanton, which, as we saw, was a solution of an Euclidean version of general relativity and electromagnetism. The solution that they found describes the moment of time at which two oppositely charged black holes are created. If one starts off from an empty universe, then one can imagine at some point the appearance of this instanton (with low probability), giving birth to this pair of extremal black holes, only for them to hurtle through spacetime. There is, of course, a chance that this pair is created and then recuperated by the vacuum, constituting a pair-creation and annihilation story.
The Garfinkle-Strominger instanton, more commonly known as a Wheeler wormhole, is not a wormhole from science fiction. In other words, it is not traversable, as if one goes through one of the black holes and they are trapped in the interior but can never cross into the other. There are many ongoing theoretical investigations on how to render wormholes traversable by using exotic matter or deformations. The wormhole seems to be a very fine-tuned possibility predicted by Einstein's theory of general relativity. Not only do the charges and mass of each black hole have to be chosen so that they are extremal, but the charges themselves have to be chosen to be opposite each other. Otherwise, the process would violate the conservation of charge! However, recent work on extremal black holes has shown that it is no more difficult to produce extremal black holes in collapse models than it is in non-extremal ones. Thus, this theoretical construction that lives at the heart of gravity and quantum theory, the unification of which is called quantum gravity, may not be a curious abstraction but perhaps a real possibility for the fate of our universe.